UPSKILL MATH PLUS
Learn Mathematics through our AI based learning portal with the support of our Academic Experts!
Learn moreLet us recall that if the sum of the two acute angles is \(90^{\circ}\), then the angles are said to be complementary.
In a right-angled triangle, the sum of two acute angles are \(90^{\circ}\).
That is, we can say that the two acute angles in a right-angled triangle are complementary.
Consider the triangle \(PQR\) right-angled at \(P\).
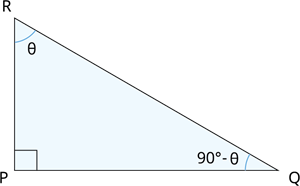
Here \(R\) and \(Q\) are complementary angles.
Therefore if \(\angle R\) \(=\) \(\theta\), then \(\angle Q\) \(=\) \(90^{\circ} - \theta\).
Let us write all the trigonometric ratios with respect to \(\angle R\) \(=\) \(\theta\) in a table.
Table \(1\):
Trigonometric ratio | Relationship with the \(\Delta PQR\) | Trigonometric ratio | Relationship with the \(\Delta PQR\) |
\(\sin \theta\) | \(\sin \theta\) \(=\) \(\frac{PQ}{RQ}\) | \(\text{cosec}\,\theta\) | \(\text{cosec}\,\theta\) \(=\) \(\frac{RQ}{PQ}\) |
\(\cos \theta\) | \(\cos \theta\) \(=\) \(\frac{PR}{RQ}\) | \(\sec \theta\) | \(\sec \theta\) \(=\) \(\frac{RQ}{PR}\) |
\(\tan \theta\) | \(\tan \theta\) \(=\) \(\frac{PQ}{PR}\) | \(\cot \theta\) | \(\cot \theta\) \(=\) \(\frac{PR}{PQ}\) |
Now let us write all the trigonometric ratios with respect to \(\angle Q\) \(=\) \(90^{\circ} - \theta\) in a table.
Table \(2\):
Trigonometric ratio | Relationship with the \(\Delta PQR\) | Trigonometric ratio | Relationship with the \(\Delta PQR\) |
\(\sin (90^{\circ} - \theta)\) | \(\sin (90^{\circ} - \theta)\) \(=\) \(\frac{PR}{RQ}\) | \(\text{cosec}\,(90^{\circ} - \theta)\) | \(\text{cosec}\,(90^{\circ} - \theta)\) \(=\) \(\frac{RQ}{PR}\) |
\(\cos (90^{\circ} - \theta)\) | \(\cos (90^{\circ} - \theta)\) \(=\) \(\frac{PQ}{RQ}\) | \(\sec (90^{\circ} - \theta)\) | \(\sec (90^{\circ} - \theta)\) \(=\) \(\frac{RQ}{PQ}\) |
\(\tan (90^{\circ} - \theta)\) | \(\tan (90^{\circ} - \theta)\) \(=\) \(\frac{PR}{PQ}\) | \(\cot (90^{\circ} - \theta)\) | \(\cot (90^{\circ} - \theta)\) \(=\) \(\frac{PQ}{PR}\) |
Comparing the tables \(1\) and \(2\), we arrive at the following identities.
1. \(\sin \theta\) \(=\) \(\cos (90^{\circ} - \theta)\)
2. \(\cos \theta\) \(=\) \(\sin (90^{\circ} - \theta)\)
3. \(\tan \theta\) \(=\) \(\cot (90^{\circ} - \theta)\)
4. \(\text{cosec}\,\theta\) \(=\) \(\sec (90^{\circ} - \theta)\)
5. \(\sec \theta\) \(=\) \(\text{cosec}(90^{\circ} - \theta)\)
6. \(\cot \theta\) \(=\) \(\tan (90^{\circ} - \theta)\)
Register for free to see more content