PDF chapter test TRY NOW
Spherical mirrors are a part of a sphere. Consider a sphere that is hollow in nature. Consider slicing a portion of a sphere and silvering the surface of the portions on the inner or outer surface, as shown in the below diagram.

If a mirror is obtained from a sphere silvered on the outer side, it is called a concave mirror.
If a mirror is obtained from a silvered sphere inside, it is called a convex mirror.
Terms related to spherical mirrors:
Length \(AB\) is the measure of aperture, \(C\) is the centre of curvature, \(P\) is the pole, and \(PC\) is the principal axis.
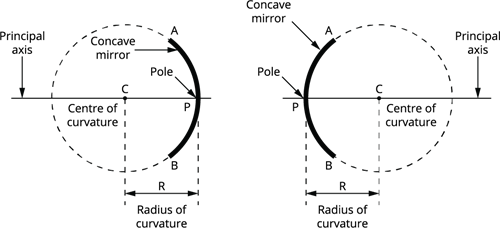
Important terms of spherical mirror
Aperture: The portion available for reflection is called aperture; \(APB\) is the aperture.
Pole: It is the geometric centre of the reflecting surface. It is denoted by \(P\).
Centre of Curvature: It is the centre of the sphere of which the mirror forms a part. \(C\) is the centre of curvature.
Principal Axis: It is a straight line passing through the centre of curvature and the pole. The line passing through \(P\) and \(C\) in the figure is the principal axis.
Radius of Curvature (\(R\)): It is the radius of the sphere of which the mirror forms a part. \(PC\) is the radius of curvature.
Principal Focus: Consider a parallel beam of light incident on a spherical mirror,
In the case of a concave mirror, the parallel beam after reflection converges at a point \(F\) which is called the principal focus.
In the case of a convex mirror, it appears to diverge from the focus (\(F\)).
Thus, a concave mirror is called a converging mirror, and a convex mirror is called a diverging mirror.
Focal Length: It is the distance between the pole and the principal focus. \(PF\) is the focal length. It is denoted by ‘\(f\)’. It is measured in \(m\) or \(cm\).
