PDF chapter test TRY NOW
There is geometry in the humming of the strings, and there is music in the spacing of spheres.
- Pythagoras
Did you know how the Thales theorem came into existence?
Thales was the most famous Greek mathematician and philosopher of the \(7^{th}\) century BC. He discovered many concepts in mathematics and astronomy. For example, when Thales was travelling around Egypt, he was challenged by the Egyptians to find the height of the Great Pyramid. Thales accepted the challenge and found the height of the Pyramid. He determined the Pyramid's height by measuring the length of its shadow.
But, when the Sun goes up, the shadow becomes short, and when the Sun is near the horizon, the shadow becomes long. To estimate the time to measure the height, Thales used a stick. When the length of the stick's shadow is equal to the stick's height, then the length of the Pyramid's shadow is equal to the height of the Pyramid.
This is how Thales determined the height of the Pyramid. To was credited for his discovery in mathematics, and the concept was named after him. It is called "Basic proportionality theorem" or "Thales theorem".
Let us consider an activity to understand the Thales theorem.
Activity:
Draw any angle \(XAY\) and mark points (say \(5\)) \(P_1\), \(P_2\), \(D\), \(P_3\) and \(B\) on the arm \(AX\) such that \(AP_1 = P_1P_2 = P_2D = DP_3 = P_3B\).
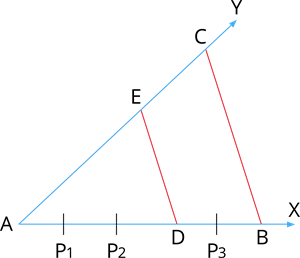
Through \(B\), draw a line intersecting the other arm \(AY\) at \(C\). Also, through \(D\), draw a line parallel to \(BC\) intersecting \(AC\) at \(E\).
Observe that, \(\frac{AD}{DB} = \frac{3}{2}\)
Also, \(\frac{AE}{EC} = \frac{3}{2}\)
Thus, in \(\triangle ABC\), \(DE \parallel BC\), then \(\frac{AD}{DB} = \frac{AE}{EC}\)
Reference:
Photo by <a href="https://unsplash.com/@stgu1610?utm_source=unsplash&utm_medium=referral&utm_content=creditCopyText">Steffen Gundermann</a> on <a href="https://unsplash.com/?utm_source=unsplash&utm_medium=referral&utm_content=creditCopyText">Unsplash</a>
Register for free to see more content