UPSKILL MATH PLUS
Learn Mathematics through our AI based learning portal with the support of our Academic Experts!
Learn moreIt is easy to understand the integers using the number line.
In the below number line, the numbers lie on the left side of the zero are negative numbers, and the numbers lie on the right side of the zero are positive numbers.
1. \(-1\), \(-100\), \(-999\), \(-9898\) are negative numbers which lies on the left side of the zero on the number line.
2. \(2\), \(90\), \(888\), \(8576\) are positive numbers which lies on the right side of the zero on the number line.
3. Zero is neither negative nor positive, and it lies at the middle.
Represent integers on a number line
- Mark the positive integers on the number line by moving its value to the right side of \(0\).
- Mark the negative numbers on the number line by moving its value to the left side of \(0\).
Example:
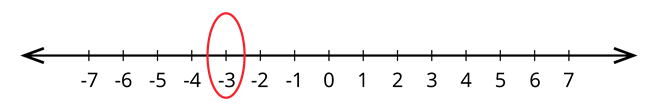
1. To mark \(-3\) on the number line, we have to move \(3\) points to the left side of zero.
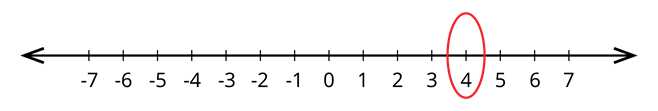
2. To mark \(4\) on the number line, we have to move \(4\) points to the right side of zero.
3. Using the number line, write the integer which is \(3\) more than \(-2\).
From \(-2\), move \(3\) units to the right side of \(-2\).
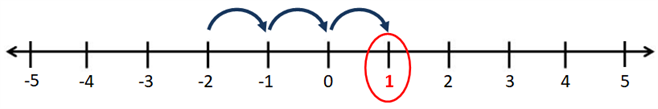
Therefore, \(3\) more than \(-2\) is \(1\).
4. Find the numbers on the number line that are at a distance of \(2\) units in the opposite directions to \(-1\).
Step 1: Here, \(-1\) is the middle term. Move, \(2\) units to the left side of \(-1\).
Step 2: Now, move \(2\) units to the right side of \(-1\).
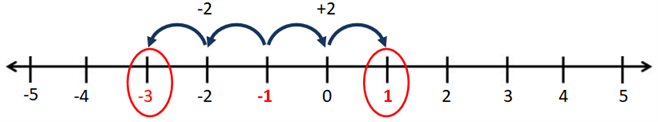
The required numbers are \(-3\) and \(1\).
Important!
\(-1\) is the greatest negative integer.
Register for free to see more content