PDF chapter test TRY NOW
The irrationals \(=\) \(3.141592653589793...\) and \(= 1.618033988749894...\)
The Greek Mathematician Archimedes was the first to compute digits in the decimal expansion of .
He discovered the value of lies between the range \(3.140845\ <\) \(<\ 3.142857\).
During (\(476\) – \(550\) C.E.) Aryabhatta, the great Indian mathematician and astronomer, found the value of correct to four decimal places \(3.1416\).
With the help of high-speed computers and advanced algorithms, \(pi\) has been computed to over \(1.24\) trillion decimal places!
Applications of \(pi\):
- In mathematics, it involves various fields like statistics, number theory and geometry. In the field of trigonometry, it is used to get the value of trigonometry function like sine, cosine, tangent...
- Formulas in the branch of sciences such as thermodynamics, mechanics and electromagnetism. It is used to measure the circular velocity of things like the wheel, motor shafts, engine parts, gears.
- To check the speed of the computer, we are finding the value of \(pi\). Because they can use it to check its accuracy.
The Golden ratio is a special number. We can get a golden ratio when we divide a line into two parts.
Consider a line segment \(AB\) and divide that into two smaller segments \(AC\) and \(CB\). Let \(AC=a\) and \(BC=b\).
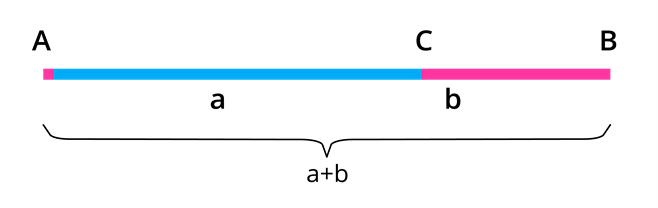
The golden ratio says the ratio of the whole line segment \(AB = a+b\) to segment \(AC = a\) is the same as the ratio of the line segment \(AC = a\) to the line segment \(BC= b\).
\(a+b : a = a : b\)
That is, .
Application of :
It appears in many branches such as art, architecture, geometry and natural science.
In the field of architecture, many beautiful monuments had constructed using the measure of the golden ratio. Here are the examples:
1. Taj Mahal, Agra:
2. The Parthenon:
3. United Nations Building, New York:
Register for free to see more content